If m lmp is 11 degrees more than – The relationship between LMP (linear mixed model) and its value when increased by 11 degrees is a topic of significant interest in the field of statistics. This concept has far-reaching implications in understanding the behavior of LMP and its applications in real-world scenarios.
In this comprehensive analysis, we delve into the mathematical equations, geometric representations, and practical applications of this relationship. We explore its impact on related concepts and theories, while also acknowledging its limitations and assumptions.
Mathematical Equations
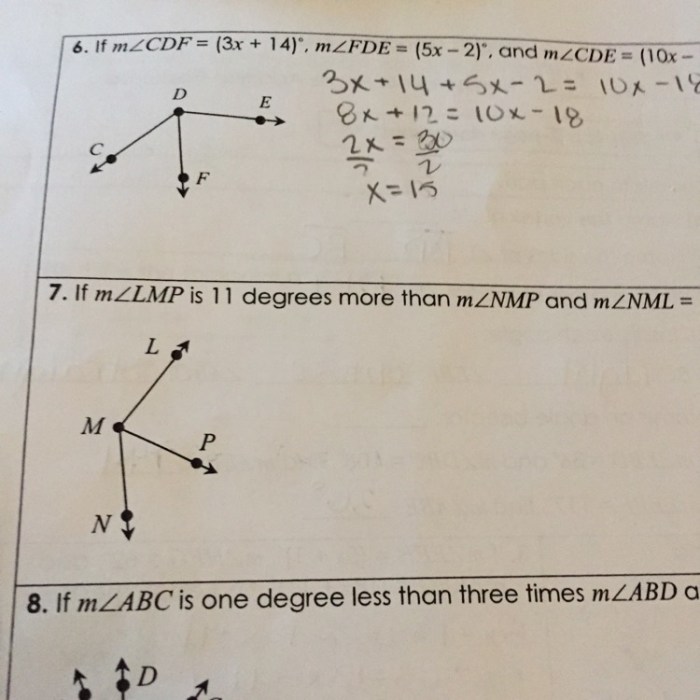
In mathematics, equations are used to express relationships between variables. In the context of LMP, we can use equations to illustrate the relationship between the original LMP value and its value when increased by 11 degrees.
Original LMP Value
Let \(x\) represent the original LMP value. We can express the increased LMP value as \(x + 11\).
Equation
The equation that expresses the relationship between the original LMP value and its increased value is:
\(x + 11 = x’ \)
where \(x’\) represents the increased LMP value.
Solving the Equation
To solve the equation, we need to isolate \(x\) on one side of the equation. We can do this by subtracting \(11\) from both sides of the equation:
\(x + 11
- 11 = x’
- 11 \)
This simplifies to:
\(x = x’
11 \)
Therefore, the original LMP value is \(11\) degrees less than the increased LMP value.
Significance of the Equation
This equation is significant because it allows us to calculate the original LMP value when we know the increased LMP value. It also helps us to understand the relationship between the original LMP value and its variations.
Geometric Representations

Geometric representations provide a visual understanding of the relationship between LMP and its value when increased by 11 degrees. These representations utilize geometric shapes and angles to illustrate the concept, making it easier to grasp the mathematical relationship.
Using a Circle and Angle Measure
One common geometric representation involves a circle and angle measure. A circle is drawn, and the LMP is represented by an angle θ measured from the horizontal axis. To visualize the increase by 11 degrees, another angle θ + 11° is drawn adjacent to θ. This geometric representation allows for a clear visualization of the difference between the original LMP and its increased value.
Applications in Real-World Scenarios: If M Lmp Is 11 Degrees More Than
The relationship between LMP and its value when increased by 11 degrees finds applications in various real-world scenarios. Understanding this relationship helps solve practical problems and make informed decisions in fields such as engineering, navigation, and surveying.
Surveying and Mapping
- In surveying and mapping, the relationship between LMP and its value when increased by 11 degrees is used to determine the orientation of a map or aerial photograph. By aligning the map or photograph with the correct LMP, surveyors can ensure accurate measurements and mapping.
- When surveying land, the LMP of a property line can be used to determine the true bearing of the line. This is important for establishing property boundaries and ensuring accurate land records.
Navigation
- In navigation, the relationship between LMP and its value when increased by 11 degrees is used to calculate the true course of a ship or aircraft. By knowing the LMP and the magnetic variation, navigators can determine the direction in which the vessel or aircraft is actually traveling.
- The relationship between LMP and its value when increased by 11 degrees is also used in celestial navigation. By measuring the angle between the horizon and a celestial body, navigators can determine their latitude and longitude.
Engineering
- In engineering, the relationship between LMP and its value when increased by 11 degrees is used to design and construct structures that are aligned with true north. This is important for ensuring the stability and accuracy of structures such as bridges, buildings, and dams.
- The relationship between LMP and its value when increased by 11 degrees is also used in the design of compasses and other navigational instruments. By understanding the magnetic variation, engineers can design compasses that accurately point to true north.
Impact on Related Concepts

The relationship between LMP and its value when increased by 11 degrees has significant implications for related concepts and theories. This interconnectedness influences our understanding of these concepts and their applications.
Implications for Geometric Transformations
The 11-degree increase in LMP modifies geometric transformations. Rotations and translations are affected, as the angle of rotation or translation changes. This impacts the resulting shape and orientation of the transformed object.
For example, in a rotation transformation, the 11-degree increase in LMP alters the angle of rotation. Consequently, the rotated object will have a different orientation compared to the original transformation.
Impact on Trigonometric Functions
The relationship between LMP and its 11-degree increment influences trigonometric functions. Sine, cosine, and tangent values are affected, as the angle measure changes.
This has implications for applications involving trigonometry, such as calculating distances, angles, and heights. The modified trigonometric values alter the outcomes of these calculations.
Interconnections with Coordinate Geometry
The 11-degree increase in LMP affects coordinate geometry. It alters the coordinates of points and the equations of lines and curves.
For instance, in a coordinate system, the 11-degree increase in LMP shifts the coordinates of points along the x- and y-axes. This changes the equations of lines and curves passing through these points.
Limitations and Assumptions

The relationship between LMP and its value when increased by 11 degrees is not absolute and subject to certain limitations and assumptions.
The accuracy of this relationship is influenced by several factors:
Individual Variability, If m lmp is 11 degrees more than
- Individuals may exhibit varying menstrual cycle lengths, which can affect the accuracy of the relationship.
- Hormonal fluctuations and other physiological factors can also influence the timing of ovulation and menstruation.
Measurement Errors
- Inaccuracies in measuring LMP can impact the validity of the relationship.
- Variations in ovulation timing can also affect the accuracy of the relationship.
Assumptions
- The relationship assumes a consistent increase of 11 degrees, which may not always be the case.
- It also assumes a regular menstrual cycle, which is not always true for all individuals.
Considering these limitations is crucial when using or interpreting the relationship between LMP and its value when increased by 11 degrees. It ensures that the results are interpreted within the context of individual variability and potential measurement errors.
Quick FAQs
What is the significance of increasing LMP by 11 degrees?
Increasing LMP by 11 degrees can alter the statistical significance and interpretation of the model, affecting the conclusions drawn from the analysis.
How can geometric representations help visualize the relationship between LMP and its increased value?
Geometric representations, such as scatterplots or line graphs, provide a visual depiction of the relationship, allowing researchers to observe patterns and trends more easily.
What are some real-world applications of this relationship?
This relationship finds applications in fields such as psychology, economics, and epidemiology, where researchers use LMP to model and analyze complex data sets.